Thermal Behavior

Chapter 7
Thermal Behavior

Thermal Behavior – describes the material’s response to the application of heat.

Heat Capacity – indicates the material’s ability to absorb heat from surroundings.
When a material absorbs heat from its environment, its temperature rises, indicating that the atomic vibrations have increased.  A measure of this is the material property of heat capacity.  This is the amount of heat required to raise the temperature of one mole (or gram-atom) of the material by one degree Kelvin:  C = Q/ΔT (units: J/mol K) where Q is the amount of heat producing a temperature change ΔT

Specific heat - same but uses mass instead of moles:  c = q/(mΔT) (units: J/kg K)

The values will depend on whether constant volume (Cv, cv) is maintained or constant pressure (Cp, cp) while the heat is being absorbed.  The difference is minor for most solids at room T or below.

The relationship between atomic vibrations and heat capacity show that at very low temperatures Cv rises sharply from zero at 0 K (Cv =AT3) and levels off above the Debye temp (θD) and approaches 3R.  θD is below room temp for most solids. (Which is why the difference between cv and cp is minor for most solids at room T or below.) This gives a good rule of thumb for the heat capacity for most materials.

 

 

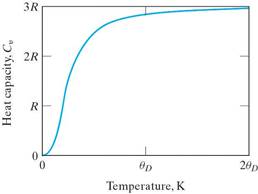
Other energy-absorbing mechanisms besides atomic vibrations that contribute to the magnitude of heat capacity are:

• Energy absorption by free electrons in metals
• Randomization of electron spins in ferromagnetic materials

 

Coefficient of Thermal Expansion
An increase of the atomic vibrations in a material will lead to an increase of the separation distance of adjacent atoms. Hence the overall dimension of the material in a given direction, L, will increase with increasing temperature.  The material property that measures this is the
linear coefficient of thermal expansion, α =   (units:  mm/mmoC) 

Note:  α will vary with temperature.

Ceramics and glasses have generally smaller values of α.  This is related to the energy wells in the bond energy diagram.  The ceramics and glasses generally have deeper energy wells, (i.e. higher bonding energies) and hence a more symmetric energy well, with relatively less increase in interatomic separation with increasing temperature. 

Young’s Modulus, E, is related to the slope of the bonding-energy curve near the bottom of the well.  The deeper the well, the larger the value of the slope and hence, larger values of E.

Some materials even have negative values of α (β-eucryptite: Li2 Al2O3 SiO2) due to atomic architecture relaxing in an accordion style as temperature increases.

Thermal Conductivity

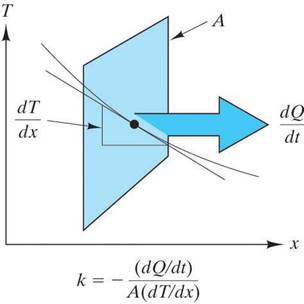Thermal conductivity, k,
is analogous to diffusivity, D.

 

Fourier’s law	Fick’s First Law
is the heat flux	is the atomic flux
The heat flux is the rate of heat transfer across an area, A, due to a temperature gradient	The atomic flux is the rate of atomic migration across an area, A, due to a concentration gradient
is the temperature gradient	is the concentration gradient
k is the proportionality constant between the heat flux and the temperature gradient.  It is the material property called thermal conductivity	D is the proportionality constant between the atomic flux and the concentration gradient.  It is the material property called Diffusivity
the units of k are J/(s m K)	the units of D are  m2/sec

 
Note:

• For steady state, the differentials become average terms.
• Like D, k is also function of T.

The conduction of heat in engineering materials involves two primary mechanisms:  atomic vibrations and conduction of free electrons. Both these mechanisms are wavelike in nature and hence will be impeded by structural disorder.  Hence, glasses have lower k than ceramics.

For ceramics, thermal energy is transported primarily by the vibrations of the atoms.  For metals, thermal energy is transported primarily by free electrons.

Hence for metals k will decrease as T ­ and as impurities ­

For ceramics and polymers k will decrease as T ­ and as amorphousness ­ and as porosity ­
(Although with some ceramics at even higher temps the k will ­ due to radiant heat x)

Thermal Shock
Thermal shock is the fracture of the material as a result of a temperature change, usually sudden cooling.  The mechanism involves both thermal expansion and thermal conductivity. 

Failures due to physical constraints:

A failure stress can be built up by constraint of uniform thermal expansion.  Consideration must be given to furnace designs and matching values of α  for coating & substrate for glazes (glass coatings on ceramics) and enamels (glass coatings on metals.)

 

 

 

 

 

Failure due to rapid temp changes which produce temporary temperature gradients (due to finite k) which result in internal residual stress: 
Consider rapid cooling of the surface of a high-temperature wall.  The surface contracts more than the interior, which is still relatively hot.  As a result, the surface “pulls” the interior into C and itself into tension.  This surface tensile stress creates the potential for brittle fracture. 

 

 

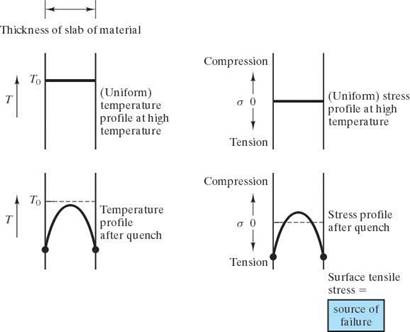
The ability of a material to withstand thermal shock depends on

• Thermal expansion coefficient
• Thermal conductivity
• Overall geometry
• Inherent brittleness
• Phase changes